算額（その1502）

算額（その1502）

六十三 花泉町金沢 大門神社・大問観世音菩薩堂 明治13年(1880)
山村善夫：現存 岩手の算額，昭和52年1月30日，熊谷印刷，盛岡市.
http://www.wasan.jp/yamamura/yamamura.html

今有如図 03064
https://w.atwiki.jp/sangaku/pages/292.html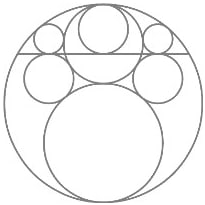

キーワード：円8個，外円，弦
#Julia, #SymPy, #算額, #和算

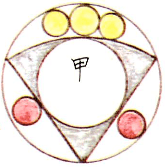
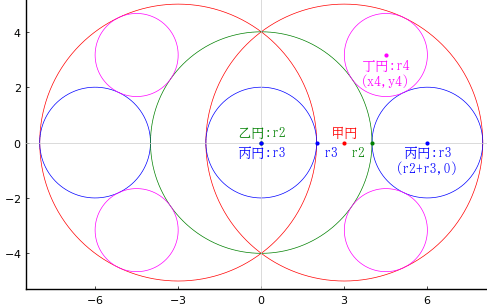
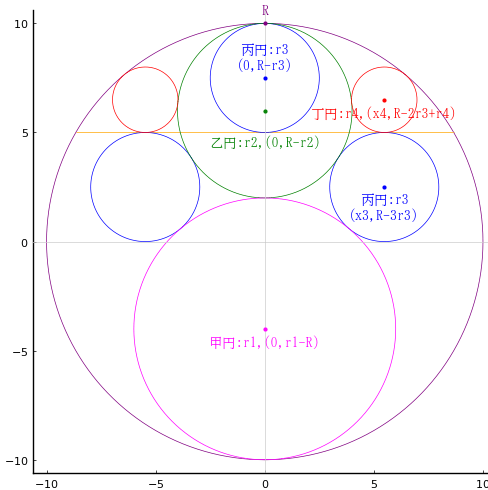
外円の中に水平な弦と甲円，乙円を容れ，隙間に丙円 3 個，丁円 2 個を容れる。甲円，乙円の直径がそれぞれ 12 寸，8 寸のとき，丁円の直径はいかほどか。

「今有如図」の図が正しい。これに基づいて得た解は，答，術に一致する。

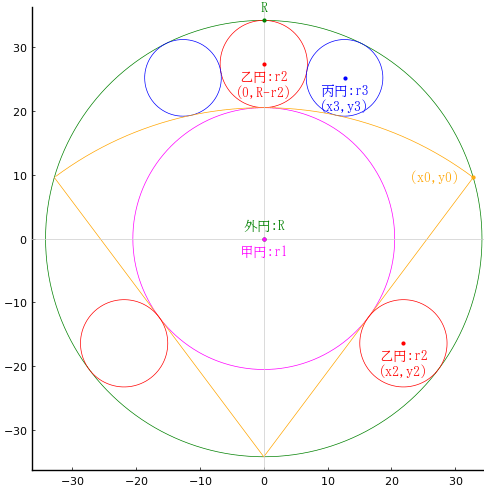
外円の半径と中心座標を R, (0, 0)
甲円の半径と中心座標を r1, (0, r1 - R)
乙円の半径と中心座標を r2, (0, R - r2)
丙円の半径と中心座標を r3, (0, R - r3), (x3, R - 3r3)
丁円の半径と中心座標を r4, (x4, R - 2r3 + r4)
とおき，以下の連立方程式を解く。

include("julia-source.txt");

using SymPy

@syms R::positive, r1::positive, r2::positive, r3::positive, x3::positive, r4::positive, x4::positive
R = r1 + r2
eq1 = x3^2 + (3r3 - r2)^2 - (r2 + r3)^2
eq2 = x4^2 + (r2 - 2r3 + r4)^2 - (r2 + r4)^2
eq3 = x3^2 + (2R - 3r3 - r1)^2 - (r1 + r3)^2
eq4 = x4^2 + (R - 2r3 + r4)^2 - (R - r4)^2
res = solve([eq1, eq2, eq3, eq4], (r3, x3, r4, x4))[1]

    (r2*(r1 + r2)/(2*r1 + r2), 2*sqrt(2)*sqrt(r1)*r2*sqrt(r1 + r2)/(2*r1 + r2), r1*r2/(2*r1 + r2), 2*sqrt(2)*sqrt(r1)*r2*sqrt(r1 + r2)/(2*r1 + r2))

丁円の半径 r4 は，甲円，乙円の半径を r1, r2 とすると r1*r2/(2*r1 + r2) である。
甲円，乙円の直径がそれぞれ 12 寸，8 寸のとき，丁円の直径は 12*8/(2*12 + 8) = 3 寸である。

術は，「丁円径 = 甲円径/(2甲円径/乙円径 + 1) 」で同じ結果を得る。

function draw(r1, r2, more=false)
    pyplot(size=(500, 500), showaxis=true, grid=false, aspectratio=1, label="", fontfamily="IPAMincho")
    (r3, x3, r4, x4) = (r2*(r1 + r2)/(2*r1 + r2), 2*sqrt(2)*sqrt(r1)*r2*sqrt(r1 + r2)/(2*r1 + r2), r1*r2/(2*r1 + r2), 2*sqrt(2)*sqrt(r1)*r2*sqrt(r1 + r2)/(2*r1 + r2))
    R = r1 + r2
    y = R - 2r3
    x = sqrt(R^2 - y^2)
    @printf("甲円，乙円の直径がそれぞれ %g, %g のとき，丁円の直径は %g である。", 2r1, 2r2, 2r4)
    plot()
    circle(0, 0, R, :purple)
    segment(-x, y, x, y, :orange)
    circle(0, R - r3, r3, :blue)
    circle2(x3, R - 3r3, r3, :blue)
    circle(0, R - r2, r2, :green)
    circle(0, r1 - R, r1, :magenta)
    circle2(x4, R - 2r3 + r4, r4)
    if more
        delta = (fontheight = (ylims()[2]- ylims()[1]) / 500 * 10 * 2) /3  # size[2] * fontsize * 2
        hline!([0], color=:gray80, lw=0.5)
        vline!([0], color=:gray80, lw=0.5)
        point(0, r1 - R, "甲円:r1,(0,r1-R)", :magenta, :center, delta=-delta)
        point(0, R - r2, "乙円:r2,(0,R-r2)", :green, :center, delta=-4delta)
        point(0, R - r3, "丙円:r3\n(0,R-r3)", :blue, :center, :bottom, delta=delta)
        point(x3, R - 3r3, "丙円:r3\n(x3,R-3r3)", :blue, :center, delta=-delta)
        point(x4, R - 2r3 + r4, "丁円:r4,(x4,R-2r3+r4)", :red, :center, delta=-delta)
        point(0, R, "R", :purple, :center, :bottom, delta=delta)
    end
end;

draw(12/2, 8/2, true)

算額（その1501）

算額（その1501）

五十八 岩手県花泉町 花泉天満宮 明治3年(1870)
山村善夫：現存 岩手の算額，昭和52年1月30日，熊谷印刷，盛岡市.
http://www.wasan.jp/yamamura/yamamura.html

今有如図 03056
https://w.atwiki.jp/sangaku/pages/167.html

キーワード：円7個，外円，円弧，弦2本
#Julia, #SymPy, #算額, #和算

外円の中に扇形を容れ，甲円 1 個，乙円 3 個，丙円 2 個を容れる。甲円の直径が 41 寸のとき，丙円の直径はいかほどか。

これも，山村の図は明らかにおかしい。接すべきものが接していないのは手描きのずなのでやむを得ないかもしれないが，扇型の上にある黄色の 3 個の円は同じ乙円ではない。中央にある円と左右の円の大きさは普通に考えれば同じではない。同じだとすれば真ん中の円が外円と扇形に接するならば，左右の円は外円と扇形に同時に接することはできない。
ということで，この算額図も「今有如図」の方が正しい。黄色の真ん中の円と下の赤い 2 この円が乙円，左右の黄色の 2 個が丙円である。そのように仮定して求めた解は，答，術に一致する。

外円の半径と中心座標を R, (0, 0)
甲円の半径と中心座標を r1, (0, 0); r1 = R - 2r2
乙円の半径と中心座標を r2, (0, R - r2), (x2, y2)
丙円の半径と中心座標を r3, (x3, y3)
扇型の半径と中心座標を (2R - 2r2), (0, -R)
とおき，以下の連立方程式を解く。

include("julia-source.txt");

using SymPy

@syms R::positive, r1::positive, r2::positive, r3::positive, x3::positive, y3::positive
R = r1 + 2r2
eq1 = x3^2 + y3^2 - (R - r3)^2
eq2 = x3^2 + (y3 + R)^2 - (r3 + (2R - 2r2))^2
eq3 = x3^2 + (R - r2 - y3)^2 - (r2 + r3)^2
eq4 = r1^2 + (R - r2)^2 - R^2
res = solve([eq1, eq2, eq3, eq4], (r2, r3, x3, y3))[1]

    (r1/3, 12*r1/41, 8*sqrt(10)*r1/41, 151*r1/123)

丙円の半径 r3 は，甲円の半径 r1 の 12/41 倍である。
甲円の直径が 41 寸のとき，丙円の直径は 12 寸である。

算額の問に答えるのはここまでである。
以下では，図を描くためのパラメータを求める。

扇型と外円の交点座標を (x0, y0)
扇型の半径と外円に接する乙円の中心座標を (x2, y2)
として加え，8 元連立方程式を解く。

@syms x0, y0, x2, y2
eq5 = x0^2 + y0^2 - R^2
eq6 = x0^2 + (y0 + R)^2 - (2R - 2r2)^2
eq7 = dist2(0, -R, x0, y0, x2, y2, r3)
eq8 = x2^2 + y2^2 - (R - r2)^2
eq7 = y2/x2*(y0 + R)/x0 + 1;

res2 = solve([eq1, eq2, eq3, eq4, eq5, eq6, eq7, eq8], (r2, r3, x3, y3, x0, y0, x2, y2))
res2[4]

    (r1/3, 12*r1/41, 8*sqrt(10)*r1/41, 151*r1/123, 8*r1/5, 7*r1/15, 16*r1/15, -4*r1/5)

甲円の直径が 41 のとき，全パラメータは以下のとおりである。

r2 = 6.83333;  R = 34.1667;  r1 = 20.5;  r3 = 6;  x3 = 12.6491;  y3 = 25.1667;  x2 = 21.8667;  y2 = -16.4

function draw(r1, more=false)
    pyplot(size=(500, 500), showaxis=true, grid=false, aspectratio=1, label="", fontfamily="IPAMincho")
    (r2, r3, x3, y3, x0, y0, x2, y2) = (r1/3, 12*r1/41, 8*sqrt(10)*r1/41, 151*r1/123, 8*r1/5, 7*r1/15, 16*r1/15, -4*r1/5)
    R = r1 + 2r2
    @printf("r2 = %g;  R = %g;  r1 = %g;  r3 = %g;  x3 = %g;  y3 = %g;  x2 = %g;  y2 = %g\n", r2, R, r1, r3, x3, y3, x2, y2)
    plot()
    circle(0, 0, R, :green)
    circle(0, R - r2, r2)
    circle2(x3, y3, r3, :blue)
    circle(0, 0, r1, :magenta)
    θ = atand(y0 + R, x0)
    circle(0, -R, 2R - 2r2, :orange, beginangle=θ, endangle=180 - θ)
    plot!([-x0, 0, x0], [y0, -R, y0], color=:orange, lw=0.5)
    circle2(x2, y2, r2, :red)
    if more
        delta = (fontheight = (ylims()[2]- ylims()[1]) / 500 * 10 * 2) /3  # size[2] * fontsize * 2
        hline!([0], color=:gray80, lw=0.5)
        vline!([0], color=:gray80, lw=0.5)
        point(0, 0, "外円:R", :green, :center, :bottom, delta=delta)
        point(0, 0, "甲円:r1", :magenta, :center, delta=-delta)
        point(0, R - r2, "乙円:r2\n(0,R-r2)", :red, :center, delta=-delta)
        point(x2, y2, "乙円:r2\n(x2,y2)", :red, :center, delta=-delta)
        point(x3, y3, "丙円:r3\n(x3,y3)", :blue, :center, delta=-delta)
        point(0, R, "R", :green, :center, :bottom, delta=delta)
        point(x0, y0, "(x0,y0)  ", :orange, :right, :vcenter)
    end
end;

draw(41/2, true)

算額（その1500）

算額（その1500）

五十八 岩手県花泉町 花泉天満宮 明治3年(1870)
山村善夫：現存 岩手の算額，昭和52年1月30日，熊谷印刷，盛岡市.
http://www.wasan.jp/yamamura/yamamura.html

今有如図 03056
https://w.atwiki.jp/sangaku/pages/167.html

キーワード：円10個
#Julia, #SymPy, #算額, #和算

交差する 2 個の甲円の 2 つの交点を通る乙円を描き隙間に丙円 3 個，丁円 4 個を容れる。丙円の直径が 4 寸のとき，丁円の直径はいかほどか。

山村は丙円を 6 個，丁円を 8 個描いているが，問との不一致をどのように思っていたのか?術の解説にもその点には触れておらず，実際に問を解いたとは思えない。山村のような図は描けないこともないが，連立方程式の本数が多くなるだろうと，手をつけないままにしていた。
これも，「今有如図」が正しく，その解釈に基づいて得られる解は答，術とも一致する。

甲円の半径と中心座標を r1, (r1 - r3, 0), (r3 - r1, 0)
乙円の半径と中心座標を r2, (0, 0)
丙円の半径と中心座標を r3, (0, 0), (r2 + r3)
丁円の半径と中心座標を r4, (x4, y4)
とおき，以下の連立方程式を解く。

include("julia-source.txt");

using SymPy

@syms r1::positive, r2::positive, r3::positive, r4::positive, x4::positive, y4::positive
eq1 = x4^2 + y4^2 - (r2 + r4)^2
eq2 = (x4 - (r1 - r3))^2 + y4^2 - (r1 - r4)^2
eq3 = (r1 - r3 - x4)^2 + y4^2 - (r3 + r4)^2
eq4 = (2r3 + r2) - (2r1 - r3)
eq5 = (r1 - r3)^2 + r2^2 - r1^2
res = solve([eq1, eq2, eq3, eq4, eq5], (r1, r2, r4, x4, y4))[1]

    (5*r3/2, 2*r3, 3*r3/4, 9*r3/4, sqrt(10)*r3/2)

丁円の半径 r4 は，丙円の半径 r3 の 3/4 倍である。
丙円の直径が 4 寸のとき，丁円の直径は 3 寸である。

function draw(r3, more=false)
    pyplot(size=(500, 500), showaxis=true, grid=false, aspectratio=1, label="", fontfamily="IPAMincho")
    (r1, r2, r4, x4, y4) = (5*r3/2, 2*r3, 3*r3/4, 9*r3/4, sqrt(10)*r3/2)
    @printf("r1 = %g;  r2 = %g;  r3 = %g;  r4 = %g;  x4 = %g;  y4 = %g\n", r1, r2, r3, r4, x4, y4)
    plot()
    circle2(r1 - r3, 0, r1)
    circle(0, 0, r3, :blue)
    circle2(2r1 - 2r3, 0, r3, :blue)
    circle(0, 0, r2, :green)
    circle4(x4, y4, r4, :magenta)
    if more
        delta = (fontheight = (ylims()[2]- ylims()[1]) / 500 * 10 * 2) /3  # size[2] * fontsize * 2
        hline!([0], color=:gray80, lw=0.5)
        vline!([0], color=:gray80, lw=0.5)
        point(r2 + r3, 0, "丙円:r3\n(r2+r3,0)", :blue, :center, delta=-delta)
        point(x4, y4, "丁円:r4\n(x4,y4)", :magenta, :center, delta=-delta)
        point(r2, 0, "r2 ", :green, :right, delta=-delta)
        point(r3, 0, " r3", :blue, :left, delta=-delta)
        point(r1 - r3, 0, "甲円", :red, :center, :bottom, delta=delta)
        point(0, 0, "乙円:r2", :green, :center, :bottom, delta=delta)
        point(0, 0, "丙円:r3", :blue, :center, delta=-delta)
    end
end;

draw(4/2, true)