算額(その638)
福島県郡山市田村町田母神地区 馬頭観世音(姉屋観世音)堂 明治11年
街角の数学 Street Wasan ~落書き帳「○△□」~ 3.四葉のクローバー
http://streetwasan.web.fc2.com/math15.5.10.html
等円 4 個とそれらの交点を通る円がある。緑色で示した部分の面積を求めよ。
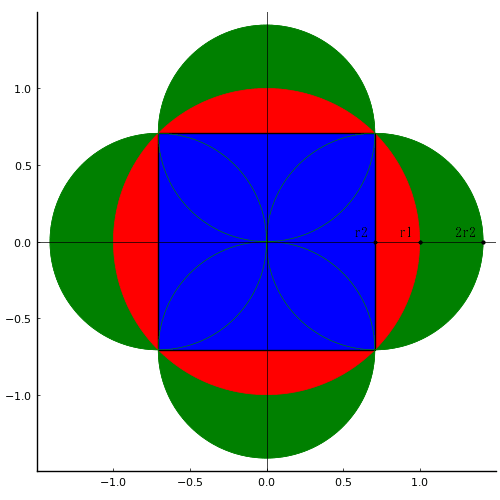
大円,等円の半径を r1,r2 とおき,図形の 1/8 (角度0度から45度まで)を考える。
include("julia-source.txt");
# julia-source.txt ソース https://blog.goo.ne.jp/r-de-r/e/ad3a427b84bb416c4f5b73089ae813cf
using SymPy
@syms r1, r2, b, r, g, x
r2 = r1/sqrt(Sym(2))
b = r2^2/2
r = PI*r1^2/8 - b
g = PI*r2^2/4 - r
println(g)
r1^2/4
緑全部の面積はこれを 8 倍したもの,すなわち大円の面積の 2 倍である。
r1 = 1 として,上部の緑色の部分の面積は,積分により以下のようになる。これを4倍して大円の面積の2倍であることが確認できる。
eq = sqrt(r2^2 - x^2) + r2 - sqrt(r1^2 - x^2)
eq = eq(r1 => 1, r2 => 1/sqrt(Sym(2)))
integrate(eq, (x, -1/sqrt(Sym(2)), 1/sqrt(Sym(2)))).evalf() |> println
0.500000000000000
function draw(more=false)
pyplot(size=(500, 500), grid=false, aspectratio=1, label="", fontfamily="IPAMincho")
r1 = 1
r2 = r1/√2
@printf("r1 = %g; r2 = %g\n", r1, r2)
plot()
circlef(0, r2, r2, :green)
circlef(0, -r2, r2, :green)
circlef(r2, 0, r2, :green)
circlef(-r2, 0, r2, :green)
circlef(0, 0, r1)
plot!([r2, r2, -r2, -r2, r2], [-r2, r2, r2, -r2, -r2], seriestype=:shape, fillcolor=:blue)
circle42(0, r2, r2, :green)
if more
delta = (fontheight = (ylims()[2]- ylims()[1]) / 500 * 10 * 2) /3 # size[2] * fontsize * 2
vline!([0], color=:black, lw=0.5)
hline!([0], color=:black, lw=0.5)
point(2r2, 0, "2r2 ", :black, :right, :bottom, delta=delta/2)
point(r1, 0, "r1 ", :black, :right, :bottom, delta=delta/2)
point(r2, 0, "r2 ", :black, :right, :bottom, delta=delta/2)
end
end;



















